The main formula for work problems is
For example, if a person can do a job in four hours, then his or her rate of work is 
A person's rate of work is the reciprocal of how long it takes to accomplish the job. Work problems are most easily done in terms of rate of work.
Example 1
George can mow a lawn in three hours. Joyce can do it in two hours. If they work together, how long will it take?
George needs three hours to do the job. Therefore, 
Joyce needs two hours to do the job. Therefore, 
Let t = the time it takes to mow the lawn together and fill in the chart (see Figure 1).
Together, they accomplish one job. Therefore,
Together, they will finish the job in 
hours, which is the same as one hour and twelve minutes. The check is left to you.
Figure 1. A chart for the problem in Example.
Example 2
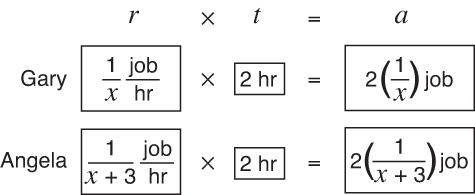
It takes Angela three hours longer to paint a fence than it takes Gary. When they work together, it takes them two hours to paint the fence. How long would it take each of them to paint the fence alone?
Let x = the number of hours it takes Gary to do the job alone.
Let x + 3 = the number of hours it takes Angela to do the job alone (Angela takes three hours longer than Gary does).
They complete the job in two hours, so fill in the chart as shown in Figure 2.
Together they finish one job: 
Therefore, x = 3 or x = –2.
Since x represents a length of time, x = –2 has no meaning in this problem. It takes Gary 3 hours and Angela 6 hours to paint the fence alone. The check is left to you.
Figure 2. A chart for the problem in Example.
