A circle is the set of points in a plane that are equidistant from one point. That one point is called the center of the circle, and the distance from it to any point on the circle is called the radius of the circle. The standard form for the equation of a circle with its center at (0, 0) and with a radius of length r is represented by the equation
x 2 + y 2 = r 2
Example 1
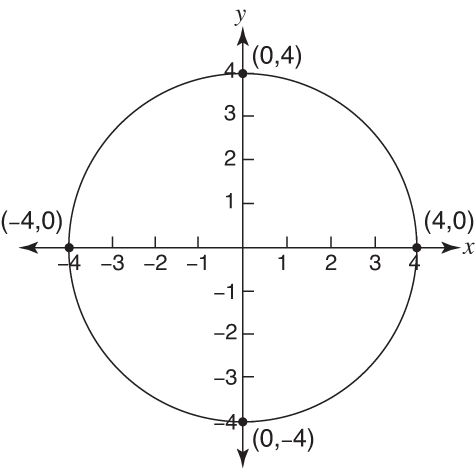
Graph x 2 + y 2 = 16.
Recognize that x 2 + y 2 = 16 is the equation of a circle centered at (0, 0) with r 2 = 16. So r = 4, as shown in Figure 1.
Figure 1. Circle in standard position.
Example 2
Find the standard form for the equation of the circle centered (0, 0) with a radius of 
.
The standard form for a circle centered at (0, 0) with a radius of r is
x 2 + y 2 = r 2
Replacing r with 
, the equation becomes 
Therefore, x 2 + y 2 = 5 is the standard form of the equation of a circle centered at (0, 0) with a radius of 
.
The standard form for a circle centered at ( h, k) with a radius of r is
( x – h) 2 + ( y – k) 2 = r 2
Note that if ( h, k) = (0, 0) that would lead to 
the equation for a circle centered at the origin.
Example 3
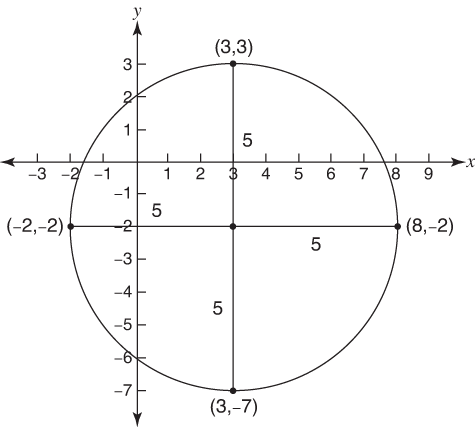
Graph the equation ( x – 3) 2 + ( y + 2) 2 = 25.
This equation represents a circle centered at (3, –2) with a radius of 
, as shown in Figure 2.
Figure 2. Circle offset down and right.
Example 4
Find the standard form for the equation of the circle centered at (–6, 2) with a radius of 
.
The standard form for the equation of a circle centered at ( h, k) with radius r is
( x – h) 2 + ( y – k) 2 = r 2
Replacing h with –6, k with 2, and r with 
, the equation becomes 
Therefore, ( x + 6) 2 + ( y – 2) 2 = 18, which is the standard form of the equation of the circle centered at (–6, 2) with radius of 
.
Example 5
From the equation given, find the center and radius for the following circle. Then graph the circle.
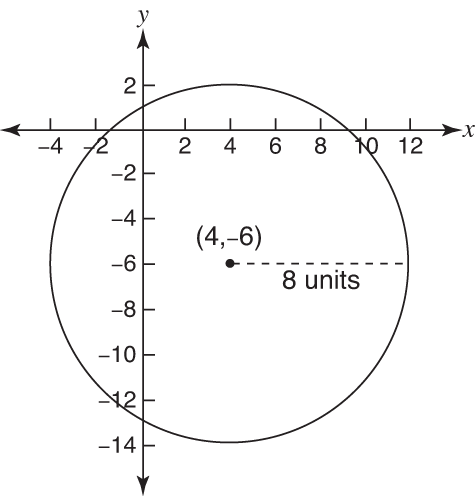
x 2 + y 2 – 8 x + 12 y – 12 = 0
This equation can be rewritten as
x 2 – 8 x + y 2 + 12 y = 12
Now, complete the square for each variable and add that amount to each side of the equation.
This circle is centered at (4, –6) with a radius of 8, as shown in Figure 3.
Figure 3. The graph of Example.
