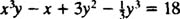A first‐order differential equation is one containing a first—but no higher—derivative of the unknown function. For virtually every such equation encountered in practice, the general solution will contain one arbitrary constant, that is, one parameter, so a first‐order IVP will contain one initial condition. There is no general method that solves every first‐order equation, but there are methods to solve particular types.
Given a function f( x, y) of two variables, its total differential df is defined by the equation

Example 1: If f( x, y) = x 2 y + 6 x – y 3, then

The equation f( x, y) = c gives the family of integral curves (that is, the solutions) of the differential equation

Therefore, if a differential equation has the form

for some function f( x, y), then it is automatically of the form df = 0, so the general solution is immediately given by f( x, y) = c. In this case,

is called an exact differential, and the differential equation (*) is called an exact equation. To determine whether a given differential equation

is exact, use the Test for Exactness: A differential equation M dx + N dy = 0 is exact if and only if

Example 2: Is the following differential equation exact?

The function that multiplies the differential dx is denoted M( x, y), so M( x, y) = y 2 – 2 x; the function that multiplies the differential dy is denoted N( x, y), so N( x, y) = 2 xy + 1. Since

the Test for Exactness says that the given differential equation is indeed exact (since M y = N x ). This means that there exists a function f( x, y) such that

and once this function f is found, the general solution of the differential equation is simply

(where c is an arbitrary constant).
Once a differential equation M dx + N dy = 0 is determined to be exact, the only task remaining is to find the function f ( x, y) such that f x = M and f y = N. The method is simple: Integrate M with respect to x, integrate N with respect to y, and then “merge” the two resulting expressions to construct the desired function f.
Example 3: Solve the exact differential equation of Example 2:
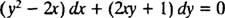
First, integrate M( x,y) = y 2 – 2 x with respect to x (and ignore the arbitrary “constant” of integration):

Next, integrate N( x,y) = 2 xy + 1 with respect to y (and again ignore the arbitrary “constant” of integration):

Now, to “merge” these two expressions, write down each term exactly once, even if a particular term appears in both results. Here the two expressions contain the terms xy 2, – x 2, and y, so

(Note that the common term xy 2 is not written twice.) The general solution of the differential equation is f( x,y) = c, which in this case becomes
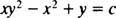
Example 4: Test the following equation for exactness and solve it if it is exact:
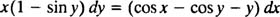
First, bring the dx term over to the left‐hand side to write the equation in standard form:

Therefore, M( x,y) = y + cos y – cos x, and N ( x, y) = x – x sin y.
Now, since

the Test for Exactness says that the differential equation is indeed exact (since M y = N x ). To construct the function f ( x,y) such that f x = M and f y N, first integrate M with respect to x:

Then integrate N with respect to y:
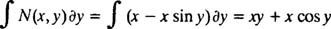
Writing all terms that appear in both these resulting expressions‐ without repeating any common terms–gives the desired function:
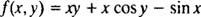
The general solution of the given differential equation is therefore
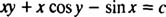
Example 5: Is the following equation exact?

Since

but

it is clear that M y ≠ N x , so the Test for Exactness says that this equation is not exact. That is, there is no function f ( x,y) whose derivative with respect to x is M ( x,y) = 3 xy – f 2 and which at the same time has N ( x,y) = x ( x – y) as its derivative with respect to y.
Example 6: Solve the IVP

The differential equation is exact because
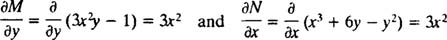
Integrating M with respect to x gives

and integrating N with respect to y yields
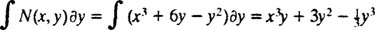
Therefore, the function f( x,y) whose total differential is the left‐hand side of the given differential equation is

and the general solution is

The particular solution specified by the IVP must have y = 3 when x = 0; this condition determines the value of the constant c:
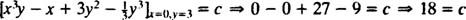
Thus, the solution of the IVP is