Solving Quadratic Equations
There are three major techniques for solving quadratic equations (equations formed by polynomials of degree 2). The easiest, factoring, will work only if all solutions are rational. The other two methods, the quadratic formula and completing the square, will both work flawlessly every time, for every quadratic equation. Of those two, the quadratic formula is the easier, but you should still learn how to complete the square.
To solve a quadratic equation by factoring, follow these steps:
1. Move all non‐zero terms to the left side of the equation, effectively setting the polynomial equal to 0.
2. Factor the quadratic completely.
3. Set each factor equal to 0 and solve the smaller equations.
4. Plug each answer into the original equation to ensure that it makes the equation true.
Example 1: Solve the equation.
Add 13 x 2and −10 x to both sides of the equation:
Factor the polynomial, set each factor equal to 0, and solve.
Because all three of these x‐values make the quadratic equation true, they are all solutions.
If an equation can be written in the form ax 2 + bx + c = 0, then the solutions to that equation can be found using the quadratic formula:
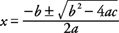
This method is especially useful if the quadratic equation is not factorable. A word of warning: Make sure that the quadratic equation you are trying to solve is set equal to 0 before plugging the quadratic equation's coefficients a, b, and c into the formula. You should memorize the quadratic formula if you haven't done so already.
Example 2: Solve the quadratic equation.
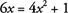
Set the equation equal to 0:
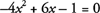
The coefficients for the quadratic formula are a = −4, b = 6, and c = −1:
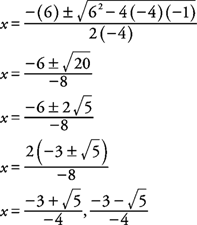
You can also write the answers as 
, the result of multiplying the numerators and denominators of both by −1. Note that the quadratic formula technique can easily find irrational and imaginary roots, unlike the factoring method.
Completing the square
The most complicated, though itself not very difficult, technique for solving quadratic equations works by forcibly creating a trinomial that's a perfect square (hence the name). Here are the steps to follow:
1. Put the equation in form ax 2 + bx = c. In other words, move only the constant term to the right side of the equation.
2. If a ≠ 1, divide the entire equation by a.
3. Add the constant value 
to both sides of the equation.
4. Write the left side of the equation as a perfect square.
5. Take the square roots of both sides of the equation, remembering to add the “±” symbol on the right side.
6. Solve for x.
Example 3: Solve the quadratic equation by completing the square.
Move the constant so it alone is on the right side:
Divide everything by the leading coefficient, since it's not 1:
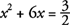
Half of the x‐term's coefficient squared, 
. Add that value to both sides of the equation:
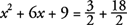
The left side is a perfect square:
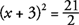
Solve for x: Don't forget that you must include a ± sign when square rooting both sides of any equation.
The answer can also be written as 
, if rationalized.