Logarithmic Functions

Whereas an exponential function answers the question “A number raised to a power equals what?” a logarithmic function (or log function) answers the question “To what power must I raise a number to get another number?” In other words, the output for a logarithmic function is in actuality an exponent.

Specifically, the logarithmic expression log _cx (read “the log base c of x”) asks the question: c to what power equals x? Thus, the equations log _cx = n and c ⁿ= x mean precisely the same thing.

Example 1: Find x in each of the equations.

log ₃ 81 = x

This expression is the equivalent of 3 ^x= 81, so x = 4. It answers the question “3 to what power equals 81?”

log ₂ x = −5

Rewrite as the equation 2 ⁻⁵ = x and evaluate; .

log _x125 = 3

Rewrite as the equation x ³ = 125 and take the cube root of each side; x = 5.

log _a 1 = x, where a is a positive integer

Rewrite as the equation a ^x= 1. No matter the value of a, only one x value will result in a value of 1: x = 0, since any positive number raised to the 0 power is 1.

Natural and common logs

Although a logarithm's base can be any positive number (except for 1, since 1 raised to any real number will still be 1), there are two bases you'll encounter most often.

Base 10. A logarithm of base 10 is called a common log. In fact, if a logarithmic expression is written without specifying a base, that base is understood to be 10, in the same way that an unwritten exponent is understood to be 1.

Base e. Just like the exponential function with base e is called the natural exponential function, the logarithm with base e is called the natural logarithm. It is used so frequently that it has its own notation: ln x, and is read “the natural log of x” or “L‐N of x,” in which case you actually say the letters L and N. Therefore, ln x is the same thing as log _ex.

Inverse relationship

Since exponential and logarithmic functions of the same base are inverses of one another, if you compose the two functions together, they will cancel one another out.

Since you will see common and natural logs most often, here is that inverse relationship expressed in terms of their respective bases:

Graphs of logarithmic functions

Since logarithmic and exponential functions are one another's inverses, it is easy to construct the graph of any logarithmic function y = log _ax based on the corresponding graph of y = a ^x. Graphs of inverse functions are reflections of one another across the line y = x, since each graph contains the coordinates of the other graph, with each coordinate pair reversed. It is no surprise, then, that because all exponential graphs of the form y = a ^xcontain the point (0,1), then all logarithmic graphs of the form y = log _ax contain the point (1,0).

In Figure , you can visually verify that the graphs of the natural logarithmic and natural exponential functions are, indeed, reflections of one another about the line y = x.

Figure 1 The graphs of y = e^xand y = in x are reflections of one another about the line y = x, as are all inverse functions.

Note that the domain of ln x, like all logarithmic functions of form y = log _ax, is (0,∞). Although it might appear that the y values of the logarithmic graph “level out,” as if approaching a horizontal asymptote, they do not. In fact, a logarithmic graph will grow infinitely tall, albeit much, much slower than its sister the exponential function. A range of (−∞,∞) for the logarithmic functions makes sense, since their inverses are exponential functions and have domains of (−∞,∞).

Change of base formula

With the aid of a scientific or graphing calculator, it is a simple matter to evaluate a logarithm. (It is not appropriate or necessary to learn to calculate complex decimal values of logarithms by hand.) However, you may notice that most computational tools have only two logarithmic buttons: one for common log and one for natural log. Thus, while it may be simple to calculate these values:

you'll need to use the change of base formula to calculate the values of logs whose base is neither 10 nor e.

According to this formula, you can rewrite a logarithm of base c as a quotient of two logs with a different base, n.

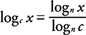
Even though you can choose any base n, you should pick either 10 or e, since that will allow you to use a calculator to find its decimal value.

Example 2: Evaluate log ₅ 9 using a calculator.

Rewrite the logarithm as a quotient of natural logs by means of the change of base formula.

You could also have rewritten log ₅ 9 as , and the final result would have been the exact same decimal value.

Precalculus