Radians

A central angle of a circle has an angle measure of 1° if it subtends an arc that is 1/360 of the circumference of the circle. This form of angle measure is quite common. Another form of angle measure that is in use is radian measure. If a central angle subtends an arc that is equal to the radius of the circle (Figure ), then the central angle has a measure of one radian.

Figure 1
Radian measure and subtended arcs

If a central angle θ of a circle with radius r subtends an arc of length q (Figure 1 ), then its radian measure is defined as

Because both q and r are in the same units, when q is divided by r in the preceding formula, the units cancel. Therefore, radian measure is unitless.

Example 1: What is the radian measure of a central angle in a circle with radius 6 m if it subtends an arc of 24 m?

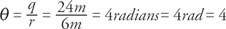
(Note that if no units are listed for an angle measure, it is assumed to be in radians.)

If θ is one complete revolution, then the subtended arc is the circumference of the circle. In this case,

Because one complete revolution is 360°,

The fact that 180° is the same as π radians is extremely important. From this relationship, the following proportion can be used to convert between radian measure and degree measure:

Example 2: What is the degree measure of 2.4 rad?

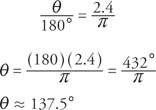
Example 3: What is the radian measure of 63°?

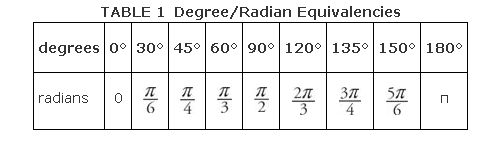
The radian measures of many special angles follow directly from the radian‐degree relationships. Some of these are summarized in Table 1 .

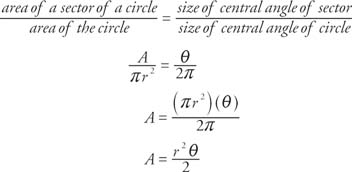
The areas of sectors of a circle are directly proportional to the measures of their central angles and directly proportional to the arcs subtended by the central angles (Figure 2 ).

Figure 2
Sector area.

Example 4: Find r given that α = 14π and θ = π/2.

Example 5: Find θ if A = 6 and r = 4.

Example 6: What is the angle measure, in radians, of the acute angle formed by the minute and hour hands of a clock at 7:15?

The hour hand moves 1/12 of a complete revolution each hour. Therefore, every 15 minutes (one quarter of an hour), the hour hand moves 1/48 of a complete revolution. Therefore, at 7:15, the hour and minute hands are 17/48 of a revolution apart.